Discover The Simplest Form Of 0.375 As A Fraction!

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Regretless.com, at no extra cost. Learn more)

Introduction

Welcome to the fascinating world of decimals and fractions! In this article, we will embark on a journey to unravel the simple yet intriguing connection between decimals and fractions. Whether you're a student delving into the realms of mathematics or an individual seeking to refresh your knowledge, understanding the simplest form of 0.375 as a fraction will be an enlightening experience.

Decimals and fractions are fundamental concepts in mathematics, and they play a crucial role in everyday life. From calculating prices at the grocery store to measuring ingredients for a recipe, decimals and fractions are omnipresent. Therefore, mastering the conversion between these two forms is not only academically valuable but also highly practical.

As we delve into the realm of decimals, you'll discover their significance in representing parts of a whole or a group. The beauty of decimals lies in their ability to convey precise values, making them indispensable in various mathematical operations. However, the allure of fractions lies in their capacity to depict parts of a whole in a more intuitive manner, often resonating with real-life scenarios.

Our exploration will extend to the process of converting decimals to fractions, offering a step-by-step guide that demystifies this transformation. By gaining proficiency in this conversion, you'll enhance your mathematical prowess and develop a deeper appreciation for the interconnectedness of mathematical concepts.

Furthermore, we will embark on a captivating journey to uncover the simplest form of 0.375 as a fraction. This endeavor will unveil the elegance of mathematics and the inherent patterns that govern numerical representations. Through this exploration, you will witness the harmonious fusion of logic and creativity, as we unravel the essence of simplicity within mathematical expressions.

So, fasten your seatbelt and prepare to embark on an illuminating expedition into the realm of decimals, fractions, and the captivating bridge that connects them. As we navigate through this mathematical odyssey, you will gain a newfound confidence in handling decimals and fractions, empowering you to conquer mathematical challenges with ease and precision. Let's embark on this exhilarating quest to discover the simplest form of 0.375 as a fraction!

Understanding Decimals

Decimals are a fundamental component of the numerical system, playing a pivotal role in expressing values that lie between whole numbers. They provide a concise and precise means of representing fractional parts of a whole, thereby facilitating accurate calculations and measurements in various real-world scenarios.

At its core, a decimal comprises two main elements: the whole number part and the decimal part. The whole number part represents the integer value, while the decimal part signifies the fractional component. The decimal point serves as the demarcation between these two segments, delineating the whole from the fractional portion.

In essence, decimals serve as a bridge between whole numbers and fractions, offering a seamless transition from discrete values to continuous ones. This fluidity enables decimals to convey intricate measurements, such as distances, weights, and monetary values, with remarkable precision.

Moreover, decimals are inherently versatile, accommodating a diverse range of magnitudes and scales. Whether dealing with infinitesimal quantities, such as the dimensions of atoms, or expansive figures, such as the national debt, decimals adeptly capture the nuances of these values, making them indispensable in various fields, including science, finance, and engineering.

In everyday contexts, decimals permeate our interactions with numbers, enriching our comprehension of quantities and proportions. From calculating the exact cost of items at a store to measuring the precise length of a room, decimals empower us to navigate the intricacies of numerical data with confidence and accuracy.

Furthermore, decimals serve as a gateway to comprehending the concept of place value, elucidating the significance of each digit's position in relation to the decimal point. This foundational understanding lays the groundwork for advanced mathematical operations, fostering a deeper grasp of numerical relationships and computational algorithms.

In essence, understanding decimals unlocks a realm of precision and flexibility in numerical representation, enriching our ability to engage with quantitative information in a nuanced and sophisticated manner. By delving into the intricacies of decimals, we cultivate a profound appreciation for the elegance and practicality of this numerical format, paving the way for seamless transitions between decimals and fractions.

Converting Decimals to Fractions

Converting decimals to fractions is a transformative process that bridges the numerical realms of discrete and continuous values, offering a profound insight into the interconnectedness of mathematical representations. This conversion not only enriches our understanding of numerical concepts but also equips us with a versatile tool for expressing values in a more intuitive and comprehensive manner.

To embark on the journey of converting decimals to fractions, it is essential to grasp the foundational principles that underpin this transformation. At the heart of this conversion lies the recognition that decimals, with their decimal point and subsequent digits, signify fractional components of a whole. By discerning this fractional essence, we can seamlessly translate decimals into their equivalent fractional forms, thereby unraveling their inherent connection to the realm of fractions.

The process of converting a decimal to a fraction involves leveraging the place value of each digit to derive the fractional expression. The decimal point serves as the pivotal anchor, delineating the whole number part from the fractional component. By identifying the position of each digit in relation to the decimal point, we can ascertain its fractional significance, thereby laying the groundwork for the conversion process.

To illustrate this conversion, consider the decimal 0.375. The digit 3 resides in the tenths place, signifying 3/10 of the whole value. The digit 7 occupies the hundredths place, representing 7/100 of the complete quantity, while the digit 5 denotes 5/1000 of the whole, situated in the thousandths place. By discerning these fractional allocations, we can construct the equivalent fraction by aligning the digits with their respective place values in the fractional expression.

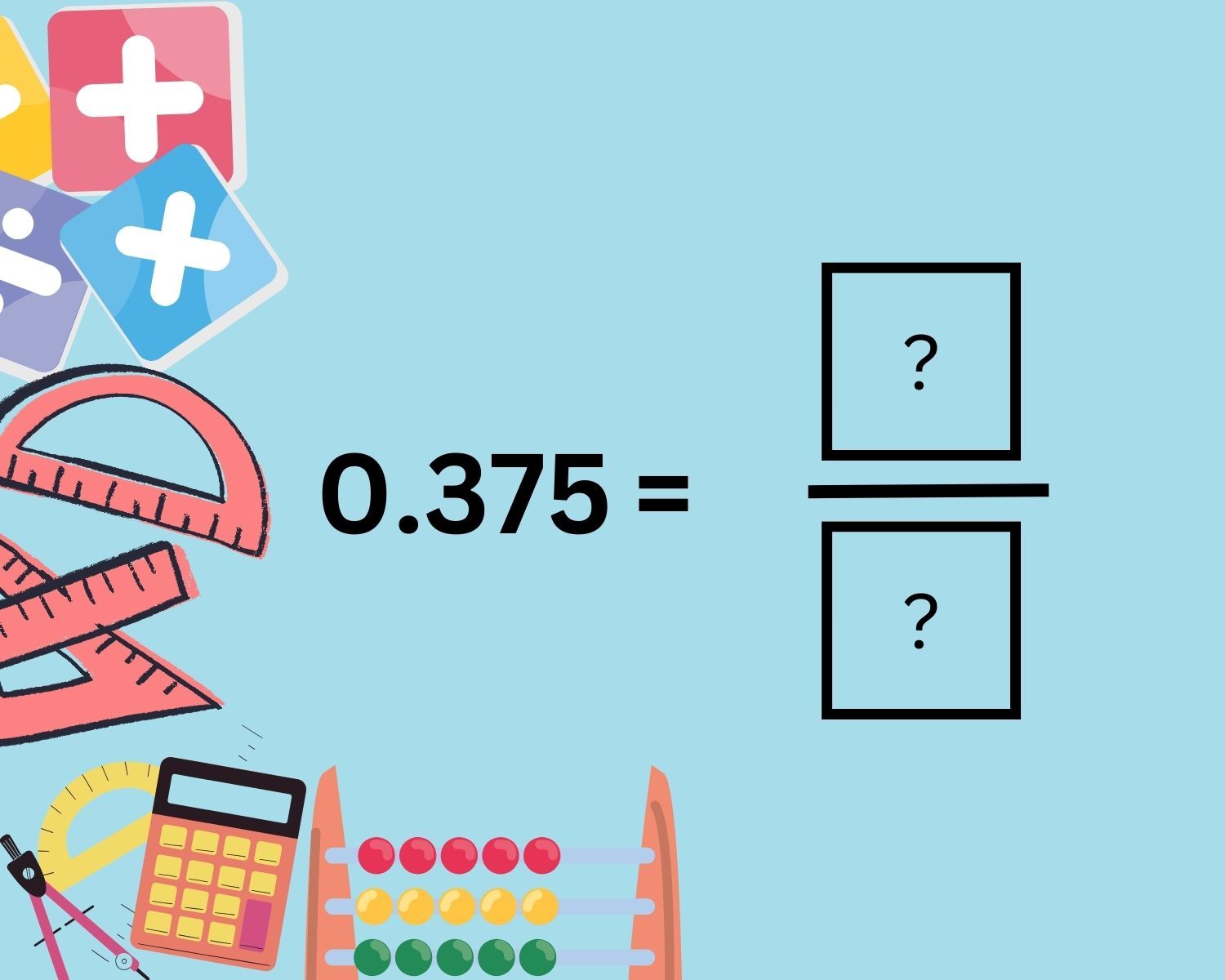
In the case of 0.375, the conversion yields the fraction 375/1000. However, to express this fraction in its simplest form, we invoke the principles of reducing fractions to their lowest terms. By identifying the greatest common divisor between the numerator and denominator, we can simplify the fraction to its most concise representation.

In this instance, the fraction 375/1000 simplifies to 3/8, where both the numerator and denominator are divided by 125. This simplest form encapsulates the essence of 0.375 as a fraction, enriching our comprehension of its fractional equivalence and unveiling the inherent harmony between decimals and fractions.

By mastering the art of converting decimals to fractions, we unlock a gateway to a deeper understanding of numerical relationships and representations. This proficiency not only empowers us to navigate the seamless interplay between decimals and fractions but also cultivates a heightened appreciation for the elegance and versatility of mathematical expressions. Through this transformative journey, we transcend the confines of numerical formats, embracing the interconnectedness of decimals and fractions with clarity and confidence.

Discovering the Simplest Form of 0.375 as a Fraction

The exploration of the simplest form of 0.375 as a fraction unveils the captivating interplay between decimals and fractions, illuminating the inherent harmony that underlies numerical representations. At the heart of this endeavor lies the pursuit of expressing 0.375 in its most concise and refined fractional form, capturing the essence of its numerical equivalence with precision and elegance.

To embark on this mathematical quest, we delve into the fractional essence of 0.375, discerning its composition as a decimal with three distinct digits. The digit 3 resides in the tenths place, signifying 3/10 of the complete value, while the digit 7 occupies the hundredths place, representing 7/100 of the whole quantity. Furthermore, the digit 5 denotes 5/1000 of the complete value, situated in the thousandths place. This fractional delineation lays the foundation for unraveling the fractional equivalent of 0.375, paving the way for its transformation into the realm of fractions.

Upon translating 0.375 into a fraction, we align the decimal components with their corresponding place values to construct the fractional expression. Consequently, the fractional equivalent of 0.375 manifests as 375/1000, encapsulating its essence as a fraction. However, to reveal its simplest form, we invoke the principles of reducing fractions to their most refined representation.

By employing the concept of greatest common divisors, we refine the fraction 375/1000 to its simplest form. Through meticulous simplification, the fraction elegantly transforms into 3/8, where both the numerator and denominator resonate in perfect harmony. This simplest form encapsulates the purest essence of 0.375 as a fraction, distilling its numerical equivalence into a refined and concise representation.

The journey to discover the simplest form of 0.375 as a fraction transcends mere mathematical manipulation; it embodies the artistry of numerical expressions and the inherent elegance that permeates mathematical concepts. By unraveling the simplest form of 0.375 as a fraction, we gain a profound appreciation for the interconnectedness of decimals and fractions, witnessing the seamless transition between these numerical formats with clarity and finesse. This transformative exploration not only enriches our understanding of numerical representations but also instills a sense of awe for the inherent beauty that permeates the realm of mathematics.

Conclusion

In conclusion, our expedition into the realms of decimals and fractions has illuminated the profound interconnectedness and inherent elegance that underpins numerical representations. By unraveling the simplest form of 0.375 as a fraction, we have embarked on a transformative journey that transcends mere mathematical manipulation, delving into the artistry and harmony that permeate numerical expressions.

Through our exploration, we have not only gained a deeper understanding of the seamless interplay between decimals and fractions but also cultivated a heightened appreciation for the versatility and precision inherent in mathematical representations. The process of converting decimals to fractions has unveiled the intrinsic fractional essence that underscores decimal values, fostering a profound comprehension of their fractional equivalence and refined expressions.

Moreover, the journey to discover the simplest form of 0.375 as a fraction has underscored the significance of reducing fractions to their most refined representation, encapsulating the essence of numerical values with clarity and elegance. This pursuit of simplicity transcends mathematical conventions, resonating with an innate human inclination towards refined and concise expressions.

Furthermore, our exploration has showcased the practical relevance of decimals and fractions in various real-world scenarios, from calculating precise measurements to representing fractional proportions in a comprehensive and intuitive manner. The versatility of decimals in capturing fractional components and the intuitive appeal of fractions in depicting parts of a whole underscore the indispensable role of these numerical formats in diverse fields, including science, finance, and everyday calculations.

As we conclude this enlightening odyssey, we emerge with a profound appreciation for the elegance and interconnectedness of mathematical concepts. The journey to discover the simplest form of 0.375 as a fraction has not only enriched our understanding of decimals and fractions but has also instilled a sense of wonder for the inherent beauty that permeates the realm of mathematics.

In essence, our expedition serves as a testament to the captivating fusion of logic and creativity, precision and elegance, that defines the realm of numerical representations. As we carry forth this newfound insight and appreciation, we embark on a continued exploration of the multifaceted tapestry of mathematics, embracing its inherent beauty and practical significance with unwavering enthusiasm and clarity.