The Surprising Fraction That Beats 1/2 And 2/3

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Regretless.com, at no extra cost. Learn more)

Introduction

Fractions are a fundamental concept in mathematics, often encountered in daily life without us even realizing it. From dividing a pizza among friends to calculating discounts during a sale, fractions play a crucial role in numerous real-world scenarios. But amidst the common fractions like 1/2 and 2/3, there exists a surprising fraction that surpasses them in a rather unexpected manner.

In this article, we will delve into the intriguing world of fractions, exploring their significance and practical applications. We will unravel the surprising fraction that outshines the commonly known 1/2 and 2/3, shedding light on its unique properties and mathematical implications. As we embark on this mathematical journey, we will uncover the hidden potential of this extraordinary fraction and its relevance in various contexts.

Join us as we unravel the mysteries of fractions and discover the remarkable characteristics of the fraction that defies conventional expectations. Through this exploration, we aim to broaden our understanding of fractions, unveiling their captivating nature and unveiling the unexpected potential of a seemingly ordinary mathematical concept.

Understanding Fractions

Fractions are a cornerstone of mathematics, representing a portion of a whole. They are a way of expressing numbers that are not whole numbers, allowing us to precisely describe quantities that fall between integers. At their core, fractions consist of two essential components: the numerator and the denominator. The numerator signifies the number of parts being considered, while the denominator represents the total number of equal parts that make up a whole.

When we encounter common fractions such as 1/2 or 2/3, we are essentially visualizing a division of a whole into equal parts. For instance, in the case of 1/2, we are dividing a whole into two equal parts and considering one of those parts. Similarly, with 2/3, we divide the whole into three equal parts and focus on two of those parts.

Fractions allow us to articulate quantities that are not neatly expressed as whole numbers. They are indispensable in various real-world scenarios, from cooking recipes that call for 1/2 cup of flour to construction plans that require 3/4 of a meter of material. Understanding fractions is pivotal for everyday tasks, as they enable us to precisely measure and distribute quantities.

Moreover, fractions serve as the foundation for more advanced mathematical concepts, including decimals, percentages, and ratios. They provide a bridge between whole numbers and more intricate numerical representations, offering a versatile and comprehensive means of expressing quantities.

In essence, fractions are not just abstract mathematical entities; they are practical tools that empower us to navigate the intricacies of measurement, comparison, and distribution in our daily lives. By comprehending the essence of fractions and their significance, we gain a deeper appreciation for their role in shaping our understanding of quantities and their relationships.

The Surprising Fraction

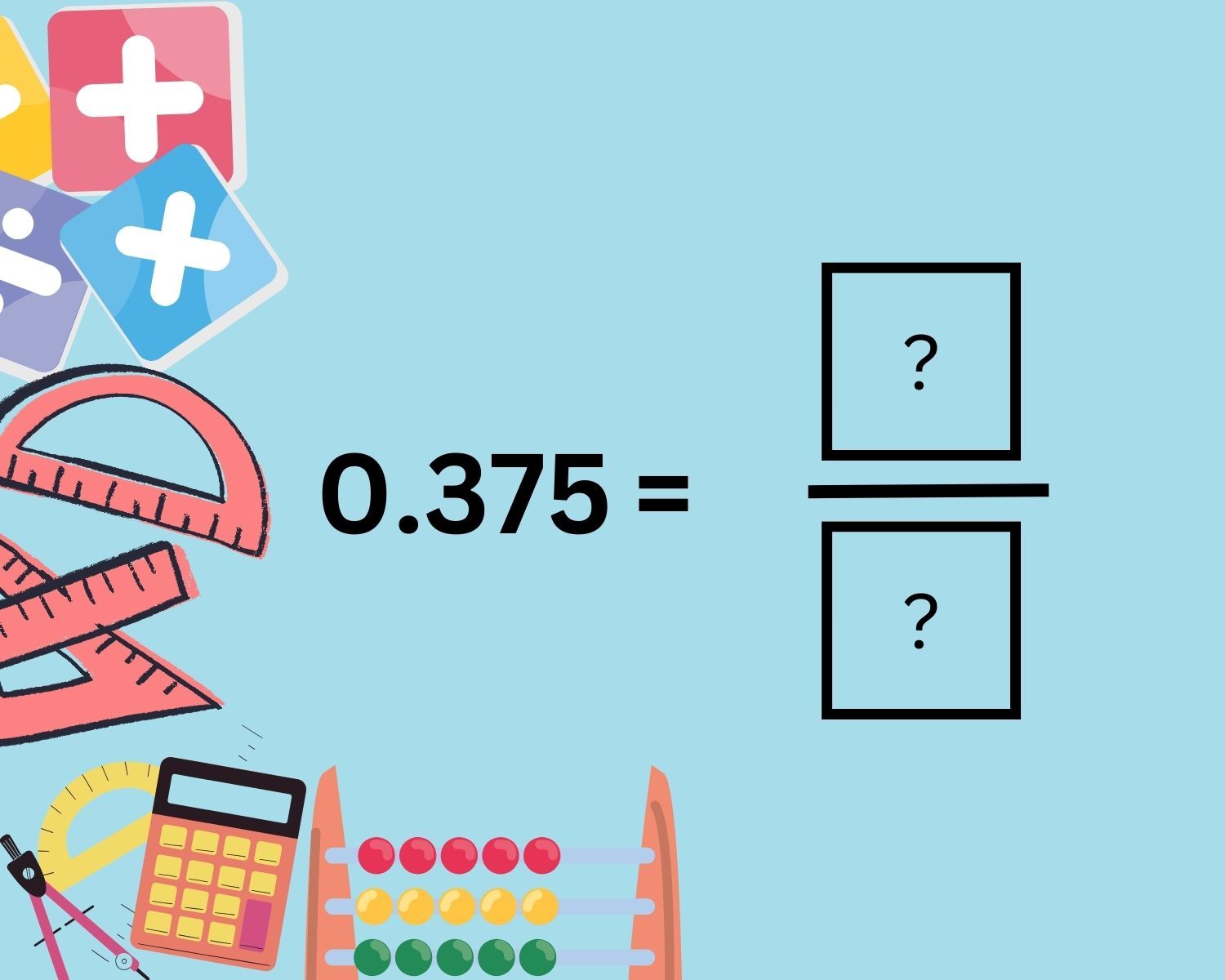
Amidst the realm of fractions, where 1/2 and 2/3 often take the spotlight, there exists a lesser-known yet remarkable fraction that defies conventional expectations. This intriguing fraction, known as 3/5, possesses unique properties that set it apart from its more renowned counterparts. While it may appear unassuming at first glance, a closer examination reveals its surprising prowess in various mathematical and practical contexts.

One of the most striking features of the fraction 3/5 is its position between 1/2 and 2/3 on the number line. This seemingly modest placement conceals a fascinating characteristic: 3/5 is actually greater than 1/2 and less than 2/3. This unexpected positioning challenges our intuitive perception of fractions, prompting us to reconsider our assumptions about their relative magnitudes.

In the realm of everyday applications, the fraction 3/5 unveils its practical significance. Consider a scenario where a group of friends decides to divide a pizza. If each person is to receive at least half of the pizza, the fraction 3/5 becomes a pivotal threshold. With 3/5 of the pizza allocated to the group, every individual is assured a portion exceeding the customary half, ensuring equitable distribution and culinary satisfaction.

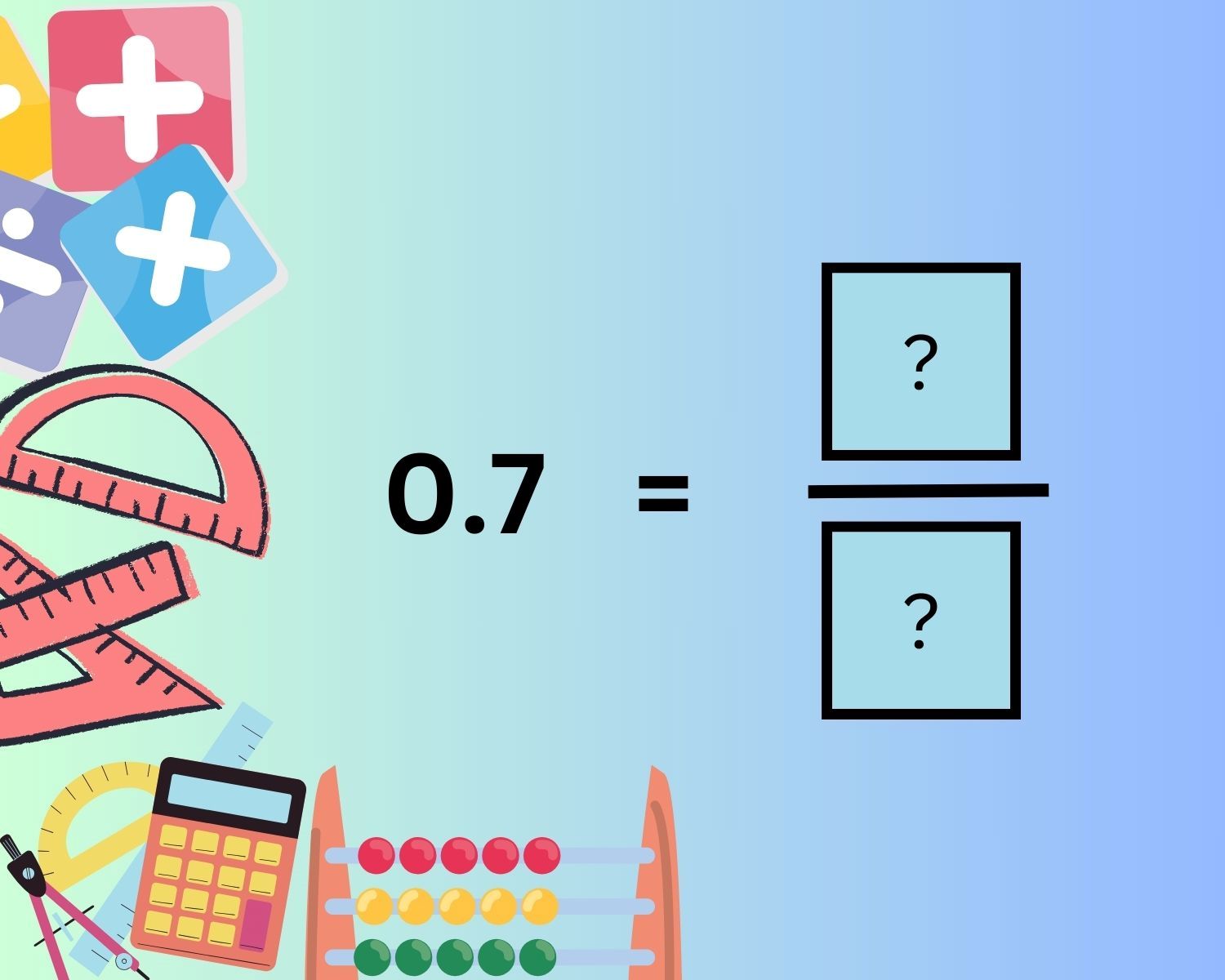
Moreover, the fraction 3/5 finds relevance in the context of percentages, offering a convenient and precise representation of 60%. This connection to percentages underscores its practical utility in numerous real-world scenarios, from calculating discounts to assessing probabilities and proportions. Its versatility as a percentage equivalent further amplifies the surprising nature of this unassuming fraction, accentuating its significance in diverse mathematical applications.

Beyond its numerical value, the fraction 3/5 embodies a compelling balance between simplicity and versatility. Its concise representation makes it easily adaptable to various computations and comparisons, while its inherent capacity to signify a substantial portion of a whole amplifies its practical appeal. This unique blend of simplicity and significance distinguishes 3/5 as a fraction that transcends its numerical identity, resonating with both mathematical precision and practical relevance.

In essence, the fraction 3/5 emerges as a captivating anomaly within the realm of fractions, challenging preconceived notions and offering a fresh perspective on numerical relationships. Its unexpected superiority to 1/2 and simultaneous inferiority to 2/3, coupled with its practical applications and versatile representation, position it as a surprising fraction that defies convention and invites us to explore the uncharted territories of mathematical intrigue.

Applications and Implications

The fraction 3/5, with its intriguing positioning between 1/2 and 2/3, holds significant applications and implications across various domains. Its unique properties and practical relevance extend far beyond numerical representation, permeating into real-world scenarios and mathematical frameworks.

In the realm of probability and statistics, the fraction 3/5 assumes a pivotal role in expressing proportions and likelihoods. When interpreting probabilities, particularly in scenarios involving discrete outcomes, the fraction 3/5 serves as a concise and intuitive representation. For instance, in a simple experiment involving the rolling of a fair six-sided die, the probability of obtaining a number less than or equal to 3 can be accurately articulated as 3/5. This direct correlation between the fraction 3/5 and favorable outcomes underscores its significance in probabilistic analyses, where clear and succinct representations are essential for informed decision-making.

Furthermore, the fraction 3/5 finds practical utility in the context of data analysis and interpretation. When evaluating proportions or percentages within datasets, the fraction 3/5 offers a straightforward and meaningful benchmark for comparison. Whether assessing survey responses, market shares, or demographic distributions, the fraction 3/5 provides a tangible reference point, enabling analysts and researchers to discern substantial segments and trends within the data. Its inherent connection to 60% further amplifies its applicability in quantitative analyses, facilitating the communication of significant findings and insights.

In educational settings, the fraction 3/5 serves as a valuable tool for fostering conceptual understanding and numerical fluency. By exploring the unique characteristics of 3/5 in comparison to 1/2 and 2/3, students can develop a deeper appreciation for the relative magnitudes of fractions and their implications in practical scenarios. Through hands-on activities, visual representations, and problem-solving tasks, educators can leverage the surprising nature of 3/5 to engage students in meaningful discussions about fractions, percentages, and real-life applications. This experiential approach not only enhances mathematical proficiency but also cultivates critical thinking skills, enabling students to discern the nuanced relationships between fractions and their broader implications.

Moreover, the fraction 3/5 resonates with economic principles and decision-making processes, particularly in the context of resource allocation and cost-benefit analyses. Whether evaluating investment returns, budget allocations, or production efficiencies, the fraction 3/5 offers a tangible reference point for assessing optimal distributions and trade-offs. Its inherent balance between substantial representation and relative moderation positions it as a compelling benchmark for evaluating the efficiency and effectiveness of resource utilization, thereby informing strategic decisions and operational optimizations.

In essence, the applications and implications of the fraction 3/5 transcend traditional numerical contexts, permeating into diverse fields and practical scenarios. Its unique positioning, practical utility, and versatile representation underscore its enduring relevance in mathematical, statistical, educational, and decision-making contexts, illuminating the unexpected depth and significance of this seemingly unassuming fraction.

Conclusion

In conclusion, the exploration of fractions, particularly the surprising fraction 3/5, has unveiled a rich tapestry of mathematical intrigue and practical significance. Through our journey into the realm of fractions, we have transcended the conventional boundaries of numerical representation, delving into the nuanced relationships and unexpected potentials inherent in these seemingly ordinary mathematical entities.

The fraction 3/5, positioned between 1/2 and 2/3, has emerged as a captivating anomaly that challenges our intuitive understanding of numerical magnitudes. Its unexpected superiority to 1/2 and simultaneous inferiority to 2/3 defies conventional expectations, inviting us to reconsider the hierarchical dynamics of fractions and their implications in diverse contexts. Beyond its numerical value, the fraction 3/5 embodies a compelling balance between simplicity and versatility, making it a formidable contender in various mathematical and practical scenarios.

Furthermore, the practical applications and implications of the fraction 3/5 extend across domains such as probability, statistics, data analysis, education, and decision-making processes. Its role as a concise and intuitive representation of proportions, likelihoods, and benchmarks underscores its enduring relevance in quantitative analyses and real-world scenarios. By leveraging the unique properties of 3/5, educators, analysts, and decision-makers can foster deeper conceptual understanding, facilitate informed interpretations, and guide strategic evaluations, thereby harnessing the inherent potential of this surprising fraction.

As we reflect on our exploration of fractions and the remarkable nature of the fraction 3/5, we are reminded of the intricate interplay between mathematical concepts and practical applications. Fractions, far from being mere abstract entities, serve as indispensable tools that empower us to navigate the complexities of measurement, comparison, and distribution in our daily lives. The unexpected prowess of the fraction 3/5 serves as a testament to the enduring allure of mathematical discovery, inviting us to embrace curiosity and delve deeper into the uncharted territories of numerical relationships and their real-world implications.

In essence, the fraction 3/5 stands as a testament to the enduring capacity of mathematics to surprise, inspire, and illuminate the intricacies of our world. By embracing the unexpected and delving into the depths of numerical intrigue, we continue to unravel the mysteries of fractions and their profound impact on our understanding of quantities, probabilities, and practical decision-making. As we navigate the boundless landscape of mathematical exploration, the fraction 3/5 beckons us to embrace the unexpected, challenge our assumptions, and uncover the hidden potentials that lie within the seemingly ordinary fabric of numbers.