Discover The Simplest Form Of 0.7 As A Fraction!

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Regretless.com, at no extra cost. Learn more)

Introduction

Fractions and decimals are fundamental concepts in mathematics, playing crucial roles in everyday calculations and problem-solving. Understanding the relationship between these two forms is essential for mastering mathematical operations and real-world applications. In this article, we will delve into the intriguing world of fractions and decimals, focusing on the process of converting the decimal representation of 0.7 into its simplest fractional form. By unraveling the steps involved in this conversion, we aim to demystify the concept of fractions and empower readers with the knowledge to tackle similar mathematical conversions with confidence and ease.

Fractions, often depicted as a numerator over a denominator (e.g., 1/2), represent parts of a whole or a collection. They are integral to various aspects of daily life, from dividing a pizza among friends to understanding financial percentages. On the other hand, decimals are a way of expressing fractions in a more compact and easily comprehensible form. The decimal representation of a fraction divides the numerator by the denominator, resulting in a finite or infinite decimal number.

In this article, we will explore the seamless interplay between fractions and decimals, shedding light on the process of converting a decimal, such as 0.7, into its fractional equivalent. This journey will not only enhance our understanding of basic mathematical concepts but also equip us with a valuable skill set for tackling mathematical challenges in diverse contexts.

As we embark on this mathematical exploration, let's embrace the beauty of numbers and the elegance of mathematical transformations. Together, we will unravel the mystery of converting 0.7 into its simplest fractional form, paving the way for a deeper appreciation of the intricate connections between fractions and decimals. So, let's dive into the world of numbers and discover the simplicity within the complexity of mathematical expressions.

Understanding Fractions

Fractions are a fundamental component of mathematics, serving as a bridge between whole numbers and decimals. At their core, fractions represent parts of a whole, allowing us to express quantities that are not whole numbers. The structure of a fraction consists of a numerator (the top number) and a denominator (the bottom number), separated by a horizontal line. The numerator signifies the number of parts being considered, while the denominator denotes the total number of equal parts that make up the whole.

When we encounter a fraction such as 1/2, it signifies that the whole has been divided into two equal parts, and we are considering one of those parts. This concept finds practical application in various real-world scenarios, such as dividing a cake into equal slices or understanding proportions in recipes.

Fractions can be categorized into different types based on their denominators. Proper fractions have numerators smaller than their denominators, such as 1/4 or 3/5. Improper fractions, on the other hand, have numerators equal to or greater than their denominators, like 7/4 or 11/3. Mixed numbers combine whole numbers with fractions, for example, 2 1/3, representing two whole units and one-third of another unit.

Understanding fractions is crucial for a multitude of mathematical operations, including addition, subtraction, multiplication, and division. Fractions also play a pivotal role in understanding percentages and ratios, making them indispensable in fields such as finance, engineering, and science.

Furthermore, fractions provide a foundation for comprehending more advanced mathematical concepts, such as algebra and calculus. They serve as building blocks for developing a deeper understanding of mathematical relationships and functions.

In essence, fractions are a cornerstone of mathematics, offering a versatile and intuitive way to express quantities that are not whole numbers. By grasping the fundamental principles of fractions, we gain a powerful tool for interpreting and manipulating numerical data, enabling us to navigate the complexities of mathematics with confidence and precision.

Converting Decimals to Fractions

Converting decimals to fractions is a fundamental skill that enhances our ability to work with diverse numerical representations. Decimals, expressed as numbers to the right of a decimal point, can be seamlessly transformed into fractions, providing a more comprehensive understanding of their underlying values. This conversion process involves translating the decimal representation into a fractional form, where the numerator and denominator reflect the original decimal's magnitude and precision.

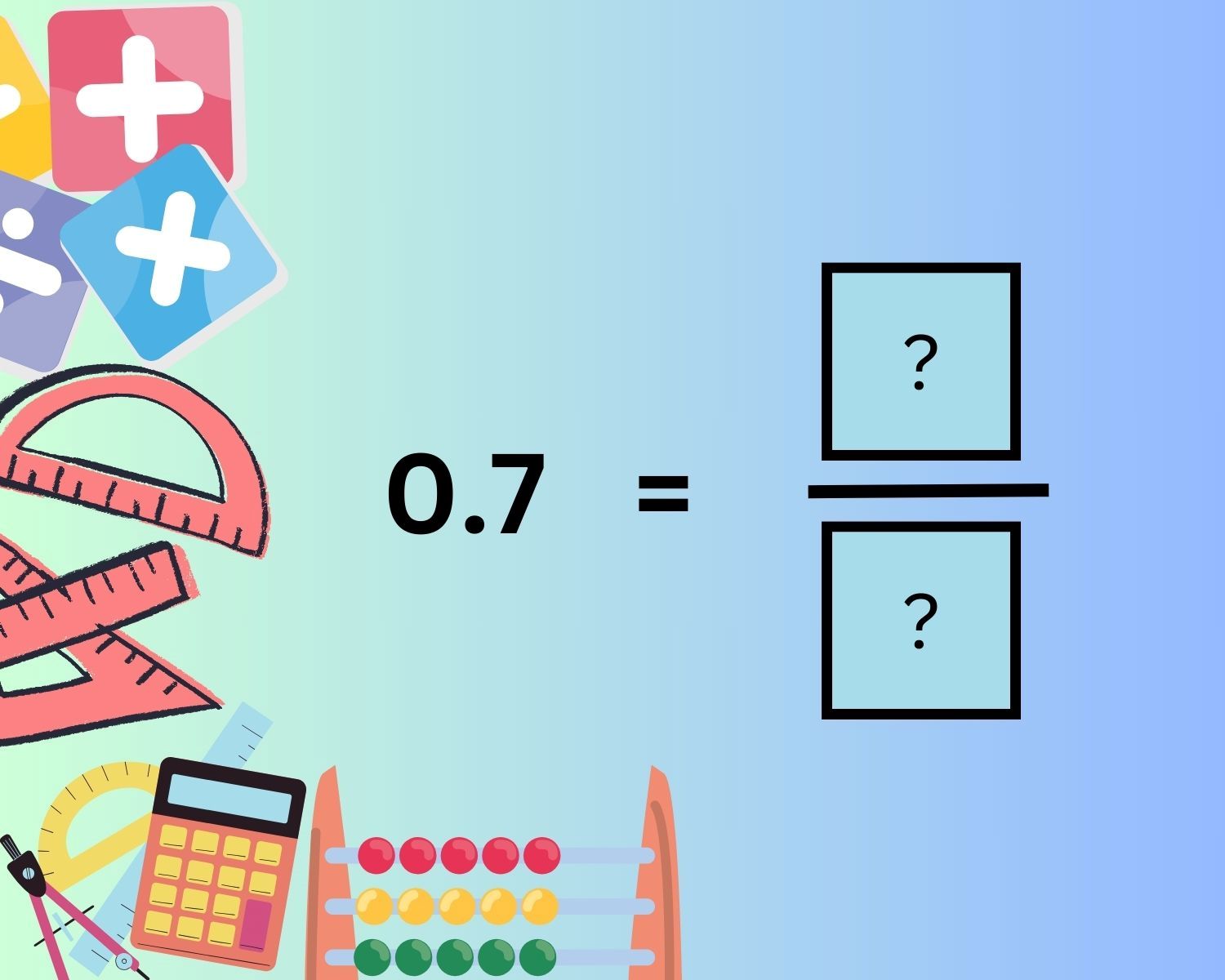
To convert a decimal to a fraction, we can leverage the relationship between decimals and place values. Each digit in a decimal holds a specific place value, such as tenths, hundredths, thousandths, and so forth. By identifying the place value of the last digit in the decimal, we can determine the fraction's denominator. For instance, in the decimal 0.7, the digit 7 is in the tenths place, indicating that the denominator of the equivalent fraction will be 10.

Once the denominator is established, the next step is to determine the numerator. This involves translating the decimal value into a whole number that represents the numerator of the fraction. In the case of 0.7, the digit 7 signifies 7 tenths, which can be expressed as 7/10. Therefore, the fractional equivalent of 0.7 is 7/10.

It's important to note that the process of converting decimals to fractions is not limited to terminating decimals (decimals that end). It also applies to repeating decimals, where one or more digits repeat infinitely. For example, the decimal 0.333… (with the digit 3 repeating infinitely) can be converted to the fraction 1/3. This showcases the versatility of the conversion process, enabling us to represent recurring patterns in decimals through concise fractional forms.

By mastering the art of converting decimals to fractions, we unlock a powerful tool for interpreting and manipulating numerical data. This skill extends our ability to seamlessly transition between different numerical representations, fostering a deeper comprehension of mathematical concepts and real-world applications. Additionally, it lays the groundwork for tackling complex mathematical problems with precision and confidence, empowering us to navigate the intricacies of decimals and fractions with ease.

In the next section, we will embark on an enlightening journey to discover the simplest form of 0.7 as a fraction, further solidifying our understanding of the interplay between decimals and fractions.

Discovering the Simplest Form of 0.7 as a Fraction

When it comes to representing decimals as fractions, the process involves translating the decimal value into a fractional form, typically expressed as a numerator over a denominator. In the case of 0.7, the decimal can be transformed into a fraction by leveraging the understanding of place value and the relationship between decimals and fractions.

The decimal 0.7 can be interpreted as 7 tenths, where the digit 7 occupies the tenths place. This insight provides a crucial clue for determining the fractional equivalent of 0.7. By recognizing that the tenths place corresponds to a denominator of 10, we can establish the foundation for expressing 0.7 as a fraction.

To convert 0.7 into a fraction, we can directly place the digit 7 as the numerator and 10 as the denominator. This yields the fraction 7/10, representing the equivalent fractional form of the decimal 0.7. In this context, the numerator (7) signifies the number of tenths, while the denominator (10) denotes the total number of equal parts that make up a whole.

The fraction 7/10 encapsulates the essence of 0.7 in its simplest form, providing a clear and concise representation of the decimal value as a fractional quantity. This process illuminates the seamless interplay between decimals and fractions, demonstrating the inherent connection between these two fundamental mathematical concepts.

Furthermore, the fraction 7/10 embodies a crucial aspect of mathematical elegance – simplicity. By expressing 0.7 as 7/10, we unveil the underlying harmony and order within seemingly complex numerical representations. This simplicity not only enhances our comprehension of mathematical relationships but also underscores the beauty of mathematical transformations.

In essence, the journey to discover the simplest form of 0.7 as a fraction unveils the intrinsic connection between decimals and fractions, showcasing the versatility and precision of mathematical representations. By embracing this process, we gain a deeper appreciation for the underlying unity of mathematical concepts, empowering us to navigate the intricacies of numerical expressions with confidence and clarity.

Conclusion

In conclusion, our exploration of converting the decimal 0.7 into its simplest fractional form has shed light on the fascinating interplay between decimals and fractions. We embarked on a journey that not only demystified the process of converting decimals to fractions but also unraveled the elegance and simplicity within mathematical representations.

Through our understanding of place value and the relationship between decimals and fractions, we seamlessly transformed the decimal 0.7 into the fractional equivalent 7/10. This process showcased the inherent connection between the two numerical forms and highlighted the precision and versatility of mathematical representations.

Moreover, the journey to discover the simplest form of 0.7 as a fraction emphasized the beauty of mathematical transformations. By expressing 0.7 as 7/10, we uncovered the underlying harmony and order within seemingly complex numerical expressions. This simplicity not only enhances our comprehension of mathematical relationships but also underscores the elegance of mathematical representations.

Our exploration also underscored the practical significance of converting decimals to fractions. This skill equips us with a valuable tool for interpreting and manipulating numerical data, fostering a deeper understanding of mathematical concepts and real-world applications. Whether in the realm of finance, engineering, science, or everyday calculations, the ability to seamlessly transition between decimals and fractions empowers us to navigate diverse mathematical challenges with confidence and precision.

As we conclude this journey, it is evident that the process of converting 0.7 into its simplest fractional form transcends mere mathematical manipulation. It embodies the essence of simplicity and elegance within the realm of numbers, enriching our perception of mathematical concepts and their interconnectedness.

In essence, our exploration has not only empowered us with the knowledge to convert 0.7 into its simplest fractional form but has also illuminated the profound beauty inherent in mathematical transformations. As we continue to unravel the mysteries of mathematics, let us carry forward this appreciation for elegance and simplicity, embracing the unity and harmony that underpin the world of numbers.

Through this journey, we have unlocked the potential to navigate the complexities of numerical representations with grace and understanding, paving the way for a deeper connection with the captivating realm of mathematics.