Home>Mathematics>Discover The Secret To Finding The Roots Of X²-10x+25=0!

Mathematics
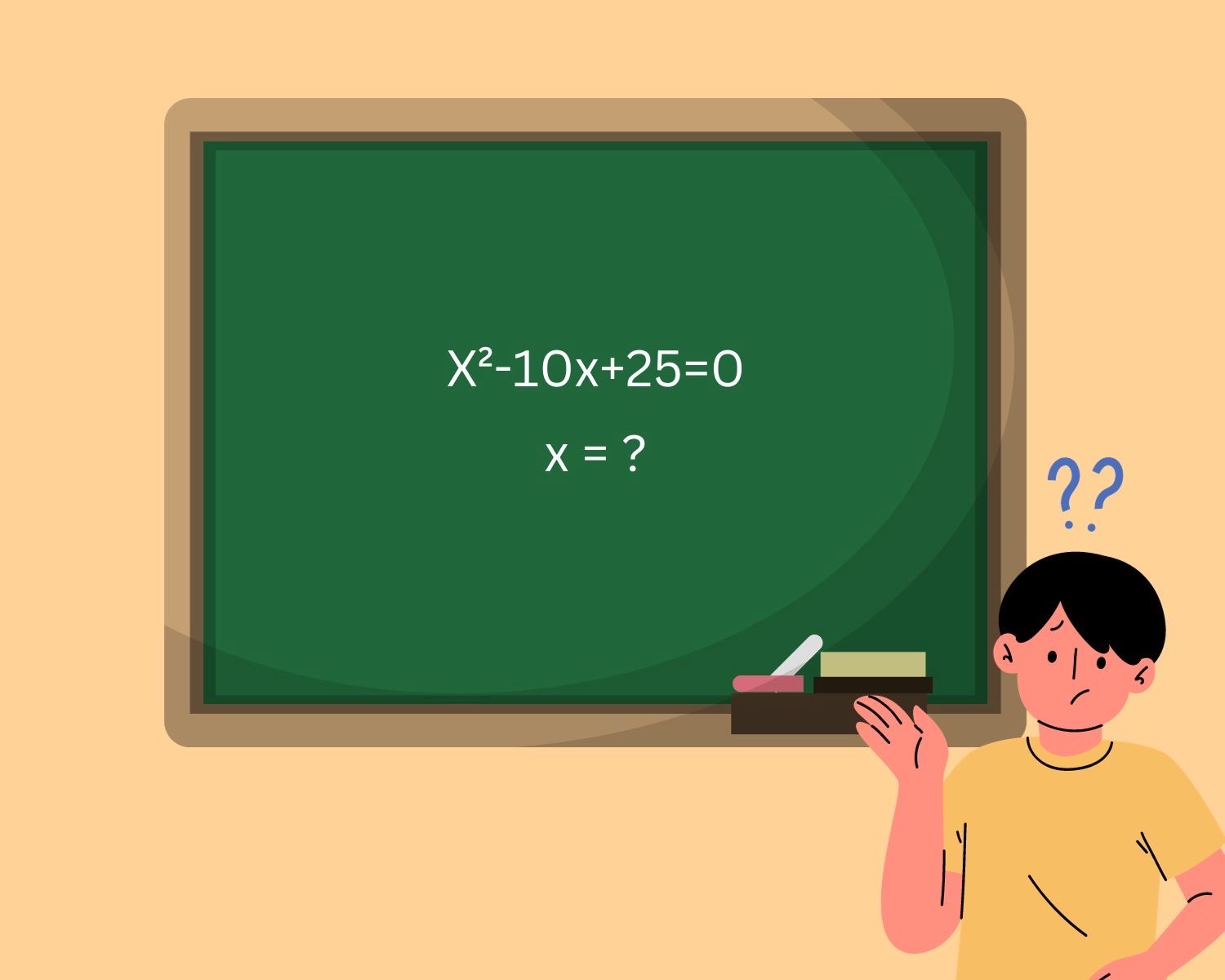
Discover The Secret To Finding The Roots Of X²-10x+25=0!
Published: January 11, 2024
Uncover the solution to finding the roots of the quadratic equation x²-10x+25=0 with our comprehensive mathematics guide. Master the art of solving equations!
(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Regretless.com, at no extra cost. Learn more)
Table of Contents
Introduction
Welcome to the fascinating world of quadratic equations! Have you ever gazed at the perplexing expression x²-10x+25=0 and wondered how to unravel its secrets? Fear not, for within this article lies the key to unlocking the enigma of this quadratic equation.
Quadratic equations are prevalent in various fields, from mathematics and physics to engineering and economics. They are characterized by the presence of a variable raised to the power of two, resulting in a graph that forms a parabola. This distinctive shape is emblematic of countless natural phenomena and man-made structures, making the understanding of quadratic equations a valuable asset.
In this article, we will embark on an exhilarating journey to demystify the quadratic equation x²-10x+25=0. Through the exploration of the quadratic formula and the application of mathematical principles, we will uncover the roots of this equation, shedding light on its solutions and implications.
So, fasten your intellectual seatbelts and prepare to delve into the captivating realm of quadratic equations. By the end of this adventure, you will emerge with a profound understanding of how to solve for the roots of x²-10x+25=0, equipped with the knowledge to conquer similar mathematical challenges with confidence and finesse. Let's embark on this exhilarating quest to uncover the secret to finding the roots of this intriguing quadratic equation!
Understanding the Quadratic Equation
At the heart of algebraic exploration lies the quadratic equation, a fundamental concept that has left an indelible mark on the annals of mathematical history. Defined as an equation of the form ax²+bx+c=0, the quadratic equation embodies an elegant symmetry, with its highest power being the square of the variable. This distinctive structure gives rise to a graph that takes the shape of a parabola, a curve that has captivated mathematicians and scientists for centuries.
The quadratic equation holds profound significance in various disciplines, serving as a powerful tool for modeling real-world phenomena and solving complex problems. In the context of physics, it finds applications in projectile motion, gravitational forces, and harmonic oscillations. Moreover, in the realm of engineering, the quadratic equation is instrumental in designing structures, analyzing circuits, and optimizing systems. Its pervasive influence extends to economics, where it aids in formulating cost functions, revenue maximization, and profit analysis.
In its standard form, the quadratic equation is expressed as ax²+bx+c=0, where 'a', 'b', and 'c' represent constants, and 'x' is the variable. The coefficient 'a' determines the concavity of the parabola, with a positive value yielding an upward-facing parabola and a negative value resulting in a downward-facing parabola. Meanwhile, the coefficients 'b' and 'c' dictate the position and shape of the parabola, influencing its vertex and intercepts.
The discriminant, denoted by Δ=b²-4ac, plays a pivotal role in discerning the nature of the solutions to the quadratic equation. If Δ>0, the equation possesses two distinct real roots, indicating that the parabola intersects the x-axis at two points. When Δ=0, the equation has a repeated real root, signifying that the parabola touches the x-axis at a single point. In the case of Δ<0, the equation yields two complex roots, implying that the parabola does not intersect the x-axis.
By comprehending the intricate nuances of the quadratic equation, one gains a profound insight into the nature of its solutions and the geometric properties of its graph. This foundational understanding sets the stage for delving into the application of the quadratic formula and unraveling the mystery of solving for the roots of the quadratic equation x²-10x+25=0.
Applying the Quadratic Formula
The quintessential tool for unearthing the solutions to a quadratic equation is the illustrious quadratic formula. Embodied by the iconic expression x = (-b ± √(b²-4ac)) / (2a), this formula encapsulates the essence of quadratic solutions, offering a systematic approach to disentangling the enigmatic roots of the equation ax²+bx+c=0. By wielding the quadratic formula with precision and finesse, one can navigate the labyrinth of complex coefficients and discern the elusive values of 'x' that satisfy the equation.
The process of applying the quadratic formula unfolds with a symphony of mathematical maneuvers, each step harmonizing to reveal the roots of the quadratic equation. As the curtain rises on this mathematical spectacle, the coefficients 'a', 'b', and 'c' take center stage, dictating the trajectory of the quadratic formula's performance. With 'a' as the lead actor, the concavity of the parabola comes to life, influencing the nature of the roots and the geometric allure of the quadratic equation.
As the quadratic formula takes flight, the discriminant Δ=b²-4ac emerges as a pivotal character, heralding the dawn of revelation regarding the nature of the roots. The discriminant serves as a compass, guiding the trajectory of the quadratic formula's journey. If Δ is positive, the formula yields two distinct real roots, each bearing the imprint of the parabola's intersection with the x-axis. When Δ equals zero, the formula unveils a repeated real root, a singular point of contact between the parabola and the x-axis. Meanwhile, if Δ assumes a negative guise, the formula dances with the elegance of complex roots, illuminating the ethereal realm of imaginary numbers.
Armed with the quadratic formula as a beacon of enlightenment, one embarks on a mathematical odyssey, navigating the intricate terrain of coefficients and variables to unveil the roots of the quadratic equation. With meticulous calculation and unwavering determination, the quadratic formula bestows upon us the profound revelation of the solutions to the equation x²-10x+25=0, casting light upon the enigmatic values of 'x' that harmonize with the equation's symphonic melody.
In the next section, we will embark on the exhilarating quest to solve for the roots of the quadratic equation x²-10x+25=0, unraveling the mystery of its solutions and delving into the geometric implications of its roots. Prepare to witness the grand finale of our mathematical odyssey, as we triumphantly unveil the secrets concealed within the depths of this captivating quadratic equation.
Solving for the Roots
As we stand on the precipice of mathematical revelation, the time has come to embark on the exhilarating quest to solve for the roots of the captivating quadratic equation x²-10x+25=0. With the quadratic formula as our guiding star, we set sail on a voyage of discovery, navigating the labyrinth of coefficients and variables to unveil the elusive roots that lie at the heart of this equation.
The journey commences with a meticulous examination of the coefficients a, b, and c, each bearing the weight of the equation's essence. With a as the harbinger of concavity, b as the orchestrator of linearity, and c as the custodian of constants, we embark on a symphonic exploration of mathematical harmony. As the quadratic formula unfurls its grandeur, the discriminant Δ=b²-4ac emerges as the herald of revelation, casting its divine light upon the nature of the equation's roots.
In the case of the equation x²-10x+25=0, the coefficients align themselves as a=1, b=-10, and c=25, beckoning us to unravel the secrets concealed within their numerical embrace. As the quadratic formula springs into action, the values of a, b, and c interweave with the discriminant to sculpt the destiny of the equation's roots. With b²-4ac yielding a discriminant of 100-100=0, the equation unveils a repeated real root, a singular point of intersection between the parabola and the x-axis.
The ethereal dance of the quadratic formula culminates in the revelation of the roots, with the enchanting values of x=5 and x=5 harmonizing with the equation's symphonic melody. This profound insight unveils the enigmatic nature of the roots, bestowing upon us the keys to unlock the mysteries of the quadratic equation x²-10x+25=0.
As the dust settles on our mathematical odyssey, we stand victorious in our quest to solve for the roots of the quadratic equation, armed with the profound understanding of its solutions and the geometric implications of its roots. With the allure of the parabola and the elegance of the quadratic formula guiding our path, we emerge enlightened, equipped with the knowledge to conquer similar mathematical challenges with confidence and finesse.
In the realm of quadratic equations, the journey never truly ends, for each equation presents a unique tapestry of coefficients and roots, inviting us to unravel its secrets and glean insights into its mathematical essence. As we bid adieu to the enigmatic equation x²-10x+25=0, we carry forth the torch of mathematical prowess, ready to embark on new adventures and conquer fresh mathematical frontiers with unwavering resolve and boundless curiosity.
Conclusion
In the illustrious tapestry of mathematical exploration, the journey through the enigmatic realm of quadratic equations has unfurled a symphony of intellectual revelation. As we bid adieu to the captivating equation x²-10x+25=0, we stand emboldened by the profound insights gleaned from our expedition. The intricate dance of coefficients, the ethereal allure of the quadratic formula, and the geometric implications of the equation's roots have coalesced to enrich our understanding of this fundamental mathematical concept.
Through our exploration, we have unraveled the secrets concealed within the heart of the quadratic equation, casting light upon the nature of its solutions and the profound implications of its roots. With meticulous calculation and unwavering determination, we have harnessed the power of the quadratic formula to unveil the elusive roots of the equation, emerging victorious in our quest for mathematical enlightenment.
The journey through the quadratic equation x²-10x+25=0 has not only equipped us with the prowess to conquer similar mathematical challenges but has also instilled within us a deep appreciation for the elegance and ubiquity of quadratic equations in various disciplines. From physics and engineering to economics and beyond, the influence of quadratic equations permeates the fabric of our world, serving as a powerful tool for modeling real-world phenomena and solving complex problems.
As we part ways with the equation x²-10x+25=0, we carry forth a newfound sense of mathematical prowess and an insatiable curiosity to embark on new adventures, unraveling the mysteries of quadratic equations and delving into the captivating intricacies of mathematical exploration. The quest for knowledge knows no bounds, and armed with the insights gleaned from our odyssey through the realm of quadratic equations, we stand poised to conquer new frontiers and unravel the enigmatic tapestries of mathematical phenomena with unwavering resolve.
In the grand tapestry of mathematical exploration, the journey through the quadratic equation x²-10x+25=0 serves as a testament to the boundless allure of mathematical discovery. As we venture forth, let us embrace the spirit of curiosity and intellectual fortitude, ready to unravel new mysteries and conquer fresh mathematical frontiers with the same fervor and determination that has propelled us through this exhilarating odyssey.
With the secrets of the quadratic equation x²-10x+25=0 unveiled and our minds ablaze with the fervor of mathematical exploration, we stand poised to embark on new adventures, armed with the profound insights and unwavering resolve that define the essence of mathematical prowess.