Discover The Secret To Determining If Quadrilateral WXYZ Is A Parallelogram!

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Regretless.com, at no extra cost. Learn more)

Introduction

Welcome to the fascinating world of quadrilaterals and the intriguing concept of parallelograms! In this article, we will delve into the secrets of determining whether a specific quadrilateral, WXYZ, qualifies as a parallelogram. By the end of this journey, you will have a profound understanding of the properties and characteristics that define a parallelogram, empowering you to confidently identify and distinguish these special quadrilaterals.

Quadrilaterals, as the name suggests, are polygons with four sides. They come in various shapes and sizes, each possessing unique attributes that set them apart from one another. Among these diverse quadrilaterals, the parallelogram holds a distinctive allure due to its symmetrical and parallel nature. The allure of the parallelogram lies in its remarkable symmetry and the intriguing relationship between its sides and angles.

As we embark on this exploration, we will unravel the essential properties of quadrilaterals and the specific criteria that define a parallelogram. Through a blend of mathematical principles and practical examples, we will demystify the process of determining whether the quadrilateral WXYZ exhibits the defining characteristics of a parallelogram.

Join me on this enlightening journey as we unlock the secrets of quadrilaterals and unravel the mystery of parallelograms. Let's embark on this mathematical adventure and uncover the hidden gems of geometric reasoning and logic. Are you ready to unveil the captivating world of quadrilaterals and unleash your prowess in identifying parallelograms? Let's dive in and unravel the enigma of WXYZ!

Understanding Parallelograms

A parallelogram is a unique and captivating quadrilateral with distinct properties that distinguish it from other geometric shapes. At the heart of a parallelogram lies a fascinating symmetry that sets it apart as a special type of quadrilateral. The defining feature of a parallelogram is the presence of two pairs of parallel sides. This characteristic symmetry gives the parallelogram a sense of balance and uniformity, making it an intriguing subject of study in the realm of geometry.

One of the fundamental properties of a parallelogram is the equality of opposite sides. This means that the opposite sides of a parallelogram are of equal length, creating a sense of equilibrium and harmony within the shape. Additionally, the opposite angles of a parallelogram are also congruent, further reinforcing its symmetrical nature. These parallel sides and congruent angles contribute to the overall balance and uniformity that define the essence of a parallelogram.

Another remarkable feature of parallelograms is the presence of diagonals that bisect each other. The diagonals of a parallelogram intersect at a point where they are divided into two equal segments. This geometric phenomenon adds to the allure of parallelograms, showcasing the precise interplay of lines and angles within the shape.

Furthermore, the properties of a parallelogram extend to its interior angles. The consecutive angles of a parallelogram, formed by the intersection of its sides, are supplementary. This means that the sum of any two consecutive angles within a parallelogram is always 180 degrees, highlighting the harmonious relationship between the angles and sides of the shape.

In essence, a parallelogram embodies a captivating blend of symmetry, balance, and uniformity, making it a captivating subject of exploration in the realm of geometry. Its defining properties, including parallel sides, congruent angles, and bisection of diagonals, contribute to the enigmatic allure of this special quadrilateral.

As we unravel the mysteries of parallelograms, we gain a deeper appreciation for the intricate relationships between lines, angles, and symmetry within geometric shapes. The profound understanding of parallelograms serves as a cornerstone for recognizing and analyzing the unique attributes of quadrilaterals, paving the way for a deeper exploration of their captivating properties and characteristics.

Properties of Quadrilaterals

Quadrilaterals, as the name suggests, are polygons with four sides. These fascinating geometric shapes exhibit a diverse range of properties and characteristics that set them apart from other polygons. Understanding the unique attributes of quadrilaterals is essential in unraveling their distinct nature and identifying specific types such as parallelograms.

One of the fundamental properties of quadrilaterals is the sum of their interior angles. Regardless of their specific type, the sum of the interior angles of any quadrilateral always amounts to 360 degrees. This intriguing mathematical principle provides a foundational understanding of the relationship between the angles within quadrilaterals, serving as a guiding principle in their analysis and classification.

Moreover, quadrilaterals showcase a remarkable diversity in their side lengths and angles. Some quadrilaterals, such as rectangles and squares, exhibit right angles, adding a sense of symmetry and balance to their structure. On the other hand, quadrilaterals like rhombuses and trapezoids possess unique combinations of side lengths and angles, contributing to their distinct geometric profiles.

Another noteworthy property of quadrilaterals lies in the classification of their sides. Quadrilaterals can be categorized based on the equality of their sides, leading to the identification of specific types such as parallelograms, trapezoids, and kites. The interplay between the lengths of their sides and the nature of their angles defines the intricate landscape of quadrilaterals, unveiling a rich tapestry of geometric diversity.

Furthermore, the diagonals of quadrilaterals play a crucial role in understanding their properties. The diagonals of certain quadrilaterals, such as kites, exhibit unique perpendicular bisecting properties, adding a layer of complexity to their geometric nature. The precise interaction of diagonals within quadrilaterals serves as a key determinant in unraveling their defining characteristics and structural intricacies.

In essence, the properties of quadrilaterals encompass a captivating array of attributes, including the sum of their interior angles, the nature of their sides and angles, and the behavior of their diagonals. Understanding these properties lays the foundation for identifying and analyzing specific types of quadrilaterals, paving the way for a deeper exploration of their unique geometric traits and the intricate relationships that define their captivating nature.

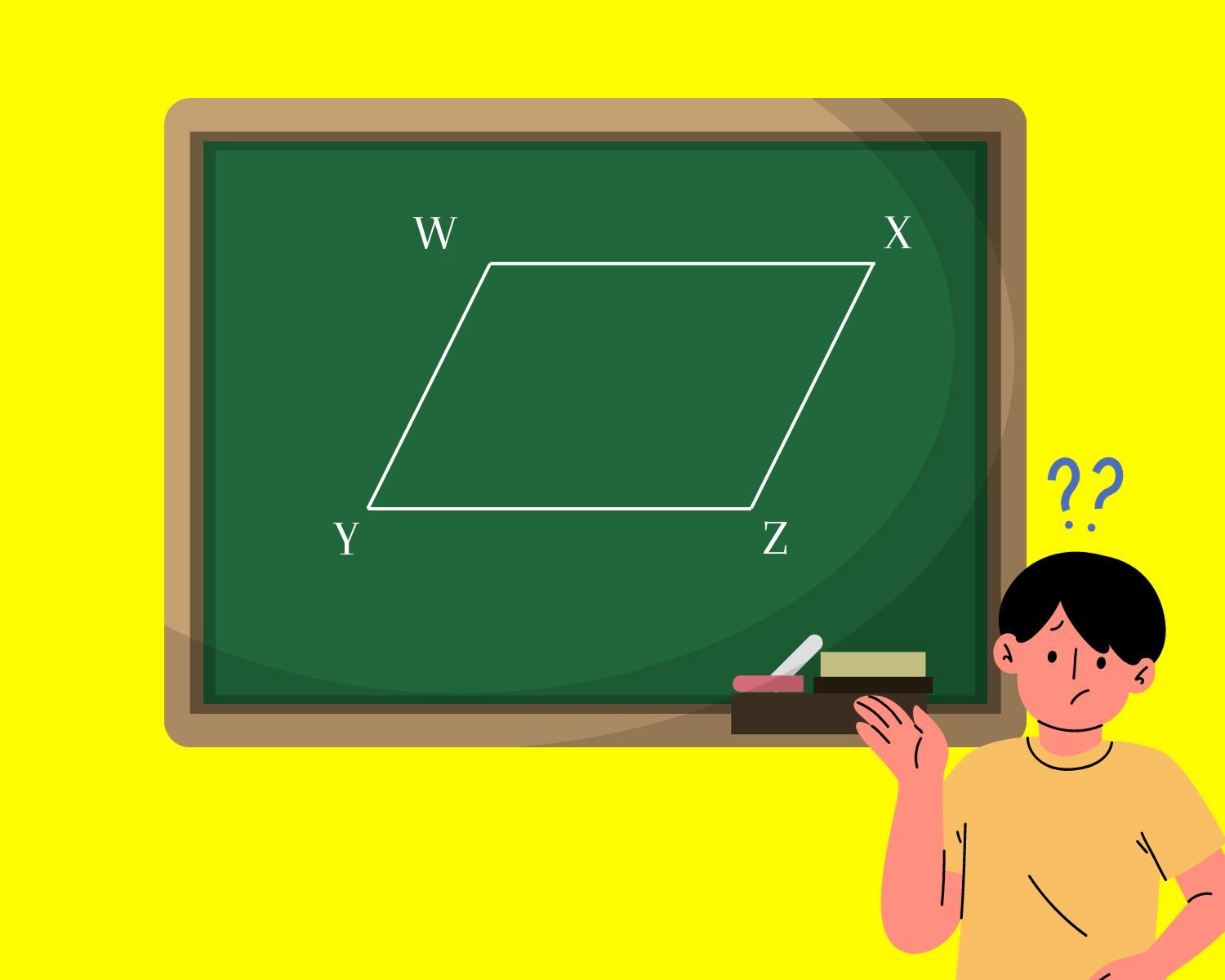
Determining if WXYZ is a Parallelogram

To determine if the quadrilateral WXYZ qualifies as a parallelogram, we must apply the essential criteria that define this captivating geometric shape. The defining feature of a parallelogram lies in the presence of two pairs of parallel sides. This fundamental characteristic sets the stage for our exploration of WXYZ and the unveiling of its geometric identity.

First and foremost, we examine the sides of quadrilateral WXYZ to ascertain the presence of parallelism. By comparing the slopes of the opposite sides, we can discern whether they are parallel, laying the groundwork for our assessment. If the slopes of the opposite sides are equal, it signifies the presence of parallelism, a crucial criterion for the classification of WXYZ as a parallelogram.

Furthermore, we delve into the lengths of the opposite sides of WXYZ, seeking equality as a hallmark of parallelograms. If the opposite sides of WXYZ are of equal length, it serves as a compelling indicator of its potential classification as a parallelogram. This equality of side lengths contributes to the symmetry and balance within the quadrilateral, aligning with the defining properties of parallelograms.

In addition to analyzing the sides of WXYZ, we explore the nature of its angles to unravel further insights into its geometric identity. By examining the measures of the consecutive angles within WXYZ, we seek to validate the presence of supplementary angles, a characteristic feature of parallelograms. The sum of consecutive angles totaling 180 degrees serves as a compelling testament to the potential parallelogram nature of WXYZ, shedding light on its geometric essence.

Moreover, we investigate the diagonals of WXYZ, exploring their potential intersection and bisection properties. The presence of diagonals that intersect at a point and bisect each other within WXYZ adds a layer of complexity to our determination, unveiling the intricate interplay of lines and angles within the quadrilateral.

As we meticulously analyze the sides, angles, and diagonals of WXYZ, we unravel the captivating intricacies of its geometric nature. Through a meticulous examination of its defining properties, including parallel sides, congruent angles, and bisection of diagonals, we gain profound insights into the potential classification of WXYZ as a parallelogram.

In essence, the process of determining if WXYZ is a parallelogram involves a meticulous exploration of its sides, angles, and diagonals, unraveling the captivating interplay of geometric properties that define its identity. By applying the essential criteria of parallelograms to our analysis, we gain a deeper understanding of the geometric essence of WXYZ, paving the way for a compelling revelation of its potential classification as a parallelogram.

Conclusion

In conclusion, the journey of unraveling the mystery of determining whether the quadrilateral WXYZ is a parallelogram has led us through a captivating exploration of geometric properties, symmetry, and the interplay of lines and angles. Our meticulous analysis of WXYZ has unveiled a rich tapestry of geometric characteristics, shedding light on the potential classification of this intriguing quadrilateral as a parallelogram.

Through our examination of the essential criteria that define parallelograms, including the presence of parallel sides, congruent angles, and bisection of diagonals, we have gained profound insights into the geometric essence of WXYZ. The interplay of its sides, angles, and diagonals has provided a compelling framework for our determination, offering a glimpse into the captivating nature of this special quadrilateral.

The process of determining the parallelogram nature of WXYZ has not only enriched our understanding of geometric shapes but has also highlighted the intricate relationships between lines, angles, and symmetry within quadrilaterals. By meticulously applying the principles of parallelograms to our analysis, we have embarked on a journey of discovery, unraveling the captivating secrets of WXYZ and its potential classification as a parallelogram.

As we conclude this exploration, we are reminded of the profound beauty and complexity inherent in the world of geometry. The enigmatic allure of quadrilaterals, particularly parallelograms, serves as a testament to the captivating nature of mathematical reasoning and logic. Our journey has not only deepened our appreciation for the geometric intricacies of WXYZ but has also honed our analytical skills in unraveling the defining properties of complex shapes.

In essence, the process of determining whether WXYZ is a parallelogram has transcended the realm of mathematical analysis, offering a glimpse into the artistry and precision that define geometric shapes. Our exploration has unveiled the captivating symmetries, balances, and harmonies within WXYZ, paving the way for a deeper understanding of the captivating world of quadrilaterals and the enigmatic allure of parallelograms.

As we conclude this mathematical odyssey, we are imbued with a profound sense of awe and appreciation for the intricate beauty of WXYZ and the tantalizing mysteries that shroud its potential classification as a parallelogram. Our journey serves as a testament to the enduring allure of geometry and the timeless quest to unravel the secrets of geometric shapes.