How To Calculate Cubic Inches

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Regretless.com, at no extra cost. Learn more)

Introduction

Cubic inches are a fundamental unit of measurement that plays a crucial role in various fields, including engineering, construction, and manufacturing. Understanding how to calculate cubic inches is essential for determining the volume of objects, which is valuable for designing, planning, and problem-solving. Whether you're a student learning about geometry or an engineer working on a new project, mastering the concept of cubic inches is a valuable skill that can be applied in real-world scenarios.

In this comprehensive guide, we will delve into the intricacies of cubic inches, exploring how they are calculated for both regular and irregular shapes. By the end of this article, you will have a solid grasp of the principles behind cubic inch calculations and how they can be applied in practical situations.

Let's embark on this journey to unravel the mysteries of cubic inches and discover the fascinating world of volume measurement. Whether you're a math enthusiast or someone seeking practical knowledge, this guide will equip you with the tools to confidently tackle cubic inch calculations for a wide range of shapes and objects. So, buckle up and get ready to explore the captivating realm of cubic inches!

Understanding Cubic Inches

Cubic inches are a fundamental unit of measurement used to quantify the volume of an object. When we talk about cubic inches, we are referring to the amount of space that a three-dimensional object occupies. This unit is particularly important in fields such as engineering, manufacturing, and construction, where precise measurements are crucial for designing and creating various structures and components.

To comprehend cubic inches, it's essential to grasp the concept of volume. Volume represents the total amount of space enclosed by a three-dimensional object. When we calculate the volume in cubic inches, we are essentially determining how many individual one-inch cubes can fit inside the object without any gaps or overlaps. This visualization helps us understand the capacity of the object in terms of cubic inches.

The formula for calculating the volume of a three-dimensional object in cubic inches varies depending on the shape of the object. For regular shapes such as cubes, rectangular prisms, and cylinders, the process is relatively straightforward, involving the multiplication of the object's length, width, and height. However, when dealing with irregular shapes, the calculation becomes more intricate, often requiring advanced geometric formulas or the use of modern technology such as 3D scanning and computer-aided design (CAD) software.

Understanding cubic inches is not only about performing mathematical calculations; it also involves developing a spatial awareness that allows us to visualize and conceptualize the volume of different objects. This spatial intelligence is invaluable in fields where precise measurements and spatial reasoning are essential for problem-solving and innovation.

In the next sections, we will explore the step-by-step process of calculating cubic inches for both regular and irregular shapes, providing practical examples to illustrate the application of these concepts in real-world scenarios. By gaining a deeper understanding of cubic inches, you will be equipped with a powerful tool for analyzing and quantifying the volume of diverse objects, paving the way for enhanced creativity and precision in your endeavors.

Calculating Cubic Inches for Regular Shapes

When it comes to calculating the volume of regular shapes in cubic inches, the process is relatively straightforward, thanks to the well-defined geometric properties of these objects. Regular shapes, such as cubes, rectangular prisms, and cylinders, have distinct characteristics that allow us to apply simple formulas to determine their volume accurately.

Cubes

Let's start with the humble cube, a three-dimensional shape with six identical square faces. To calculate the volume of a cube in cubic inches, we can use the formula V = s^3, where V represents the volume and s denotes the length of one side of the cube. By cubing the length of a side, we obtain the total number of cubic inches that the cube can hold within its boundaries. This straightforward formula simplifies the process of finding the volume of a cube, making it accessible for students and professionals alike.

Rectangular Prisms

Moving on to rectangular prisms, which are box-like structures with six rectangular faces, we can calculate their volume using the formula V = lwh, where l represents the length, w denotes the width, and h signifies the height of the prism. By multiplying these three dimensions together, we obtain the volume of the rectangular prism in cubic inches. This formula mirrors the intuitive concept of filling the prism with individual one-inch cubes, allowing us to visualize its volume in a tangible manner.

Cylinders

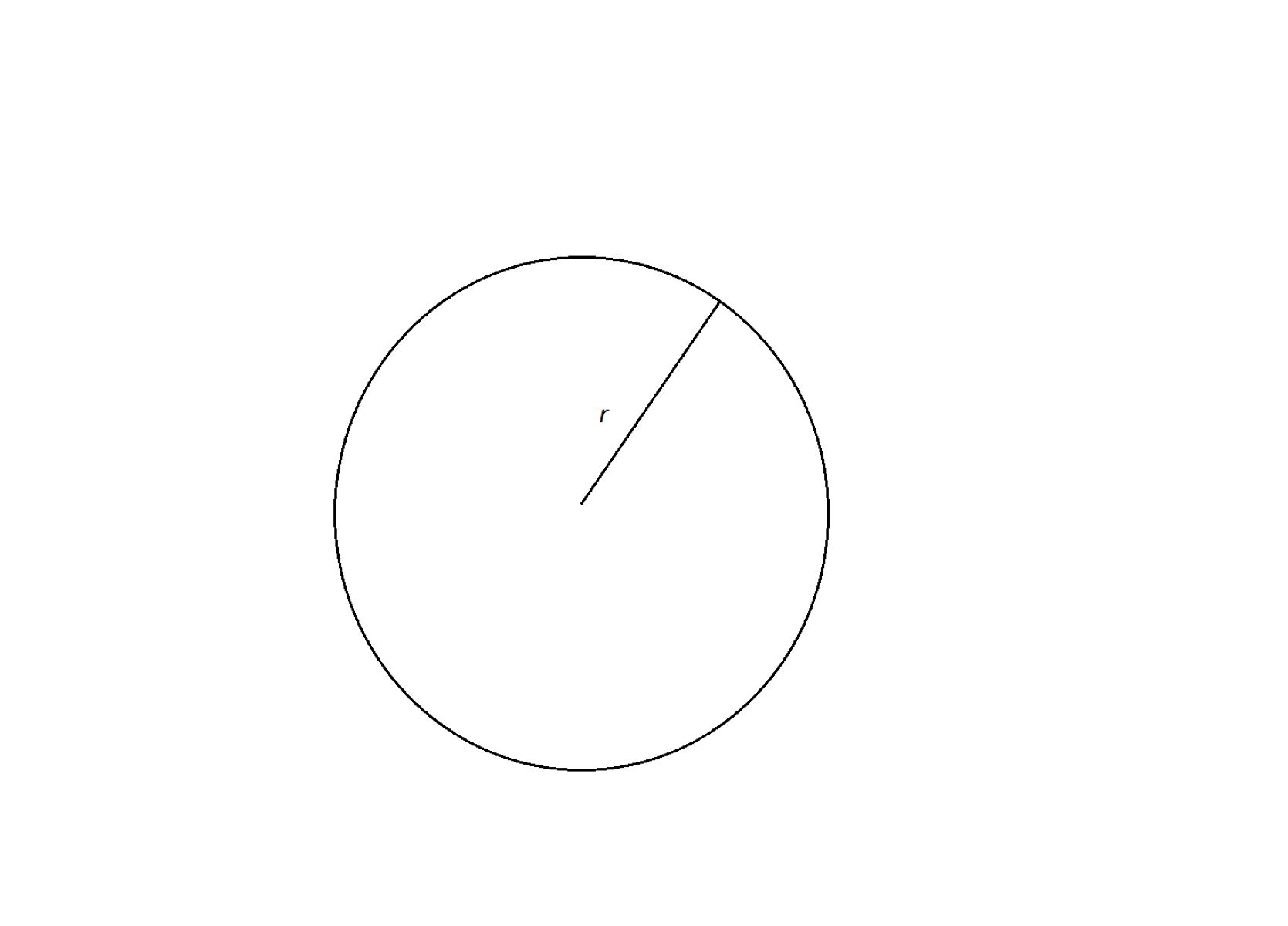
Cylinders, with their circular bases and curved surfaces, also have a simple formula for calculating their volume in cubic inches. The formula V = πr^2h, where V denotes the volume, π represents the mathematical constant pi (approximately 3.14159), r signifies the radius of the circular base, and h denotes the height of the cylinder. By multiplying the area of the base (πr^2) by the height, we can determine the volume of the cylinder in cubic inches, providing a clear understanding of its spatial capacity.

By mastering these fundamental formulas for regular shapes, individuals can confidently calculate the volume of various objects, from storage containers to architectural elements. This foundational knowledge serves as a springboard for more advanced applications, empowering learners to tackle complex volume calculations with precision and efficiency. As we delve into the realm of irregular shapes in the subsequent section, we will witness how these foundational principles can be extended to solve diverse real-world challenges, showcasing the versatility and practical significance of cubic inch calculations.

Calculating Cubic Inches for Irregular Shapes

When it comes to irregular shapes, the process of calculating cubic inches becomes more intricate due to the absence of uniform dimensions and well-defined geometric properties. Irregular shapes encompass a wide range of forms, from organic objects like rocks and tree roots to complex industrial components with asymmetrical contours. Despite their diverse and often unpredictable characteristics, it is still possible to determine the volume of irregular shapes in cubic inches through innovative approaches and advanced mathematical techniques.

One method for calculating the volume of irregular shapes involves the principle of displacement. By immersing the irregular object in a liquid and measuring the amount of liquid displaced, we can indirectly determine the object's volume. This technique, known as fluid displacement, is particularly useful for irregular objects that cannot be easily measured using traditional geometric formulas. Archimedes, the ancient Greek mathematician, famously utilized this method to determine the volume of irregularly shaped objects, laying the foundation for modern-day applications in fields such as archaeology and material science.

For irregular shapes with complex contours and varying densities, modern technologies such as 3D scanning and computer-aided design (CAD) software offer powerful solutions for calculating cubic inches. 3D scanning enables the precise capture of an object's surface geometry, allowing for the generation of a digital 3D model. By analyzing the digital model, the volume of the irregular shape can be accurately calculated using computational algorithms, providing a detailed understanding of its spatial capacity in cubic inches.

Furthermore, advanced mathematical concepts, including integral calculus and numerical methods, can be employed to calculate the volume of irregular shapes with irregular boundaries and curved surfaces. These mathematical tools enable the formulation of intricate equations that describe the shape's geometry, facilitating the precise determination of its volume in cubic inches. While these methods may require a deeper understanding of mathematical principles, they offer a robust framework for tackling the volume calculations of complex irregular shapes encountered in scientific research, engineering design, and artistic endeavors.

In the realm of practical applications, the ability to calculate cubic inches for irregular shapes is indispensable. From designing custom packaging for unique products to analyzing the volume of irregular geological formations, the knowledge and techniques for determining the volume of irregular shapes in cubic inches have far-reaching implications across various industries and disciplines.

By embracing innovative methodologies and leveraging cutting-edge technologies, individuals can navigate the complexities of irregular shapes and unlock their volumetric secrets, paving the way for enhanced creativity, problem-solving, and precision in diverse fields where irregular shapes abound.

Practical Applications of Cubic Inches Calculations

The calculations of cubic inches find extensive practical applications across diverse industries and disciplines, playing a pivotal role in shaping the physical world around us. From engineering and manufacturing to architecture and product design, the ability to accurately determine the volume of objects in cubic inches is indispensable for a wide array of real-world scenarios.

In the realm of manufacturing and production, cubic inch calculations are essential for designing and optimizing the capacity of storage containers, packaging materials, and industrial components. By precisely quantifying the volume of these objects, manufacturers can streamline their production processes, minimize material wastage, and maximize storage efficiency. Whether it's designing custom shipping crates for delicate equipment or optimizing the internal space of automotive components, cubic inch calculations empower manufacturers to make informed decisions that enhance operational efficiency and cost-effectiveness.

In the field of architecture and construction, cubic inch calculations play a crucial role in spatial planning, material estimation, and structural design. Architects and engineers rely on accurate volume measurements to determine the capacity of building elements, such as concrete foundations, steel beams, and ventilation ducts. By leveraging cubic inch calculations, professionals can optimize the allocation of space within architectural structures, ensuring that every cubic inch is utilized effectively to meet functional and aesthetic requirements.

Moreover, in the realm of product design and innovation, cubic inch calculations enable designers to create ergonomic and efficient consumer products. Whether it's designing compact electronic devices, optimizing the internal volume of household appliances, or developing custom packaging for consumer goods, the ability to calculate cubic inches is instrumental in achieving optimal product dimensions and spatial utilization. By meticulously analyzing the volume of products and their packaging, designers can enhance user experience, minimize material usage, and reduce environmental impact, aligning with the principles of sustainable design and resource efficiency.

Beyond traditional industries, cubic inch calculations also find applications in scientific research, environmental conservation, and artistic endeavors. Whether it's quantifying the volume of irregular geological formations, analyzing the spatial distribution of natural resources, or creating intricate sculptures with precise volumetric specifications, the knowledge of cubic inch calculations transcends disciplinary boundaries, offering a versatile tool for exploring and shaping the physical world.

In essence, the practical applications of cubic inch calculations are far-reaching and multifaceted, permeating various aspects of human endeavor and innovation. By harnessing the power of cubic inch calculations, individuals and organizations can unlock new possibilities, optimize resource utilization, and bring their creative visions to life in a tangible and impactful manner.

Conclusion

In conclusion, the concept of cubic inches is a fundamental pillar of measurement that permeates numerous fields, from engineering and manufacturing to architecture and artistic design. Throughout this comprehensive guide, we have delved into the intricacies of calculating cubic inches for both regular and irregular shapes, uncovering the practical significance and versatile applications of this volumetric measurement.

By understanding the principles of cubic inch calculations, individuals gain a powerful tool for analyzing, designing, and problem-solving in a three-dimensional space. The ability to determine the volume of objects in cubic inches empowers professionals and enthusiasts to optimize spatial utilization, minimize material wastage, and enhance operational efficiency across diverse industries.

From the simplicity of calculating the volume of regular shapes, such as cubes, rectangular prisms, and cylinders, to the complexity of tackling irregular shapes through innovative methodologies and advanced technologies, the journey of exploring cubic inches is a testament to human ingenuity and the quest for precision.

The practical applications of cubic inch calculations extend far beyond conventional boundaries, influencing the design of everyday products, the construction of architectural marvels, and the exploration of natural phenomena. Whether it's optimizing the internal volume of consumer electronics, designing efficient storage solutions, or analyzing the volumetric characteristics of geological formations, cubic inch calculations serve as a cornerstone for innovation and resource optimization.

As we navigate the ever-evolving landscape of technology, design, and scientific inquiry, the knowledge and skills related to cubic inch calculations continue to be indispensable. By embracing the principles outlined in this guide and leveraging modern tools and methodologies, individuals can harness the power of cubic inches to drive progress, creativity, and sustainability in a world shaped by three-dimensional possibilities.

In essence, the journey of understanding cubic inches transcends mere mathematical calculations; it embodies the spirit of exploration, problem-solving, and the relentless pursuit of precision. By mastering the art of cubic inch calculations, individuals embark on a transformative odyssey that unlocks new dimensions of creativity, efficiency, and innovation, shaping a future where every cubic inch is a canvas for human ingenuity.