The Mind-Boggling Secrets Behind Trigonometry’s Infamous Difficulty

(Many of the links in this article redirect to a specific reviewed product. Your purchase of these products through affiliate links helps to generate commission for Regretless.com, at no extra cost. Learn more)

Introduction

Trigonometry, a branch of mathematics that deals with the study of angles and the relationships between the sides and angles of triangles, has long been regarded as a formidable subject by students and educators alike. Its reputation for complexity precedes it, often evoking a mix of fascination and trepidation among those who encounter it. The mere mention of trigonometry can conjure images of intricate diagrams, perplexing equations, and bewildering theorems, leaving many to wonder about the enigmatic nature of this mathematical discipline.

As we delve into the captivating world of trigonometry, we will unravel the historical origins of this field, explore the intricacies of trigonometric functions and identities, and shed light on the real-world significance of this mathematical tool. Additionally, we will examine the unique challenges that arise in teaching and learning trigonometry, offering insights into the perplexing nature of this subject and the strategies employed to navigate its complexities.

Join us on this illuminating journey as we demystify the mind-boggling secrets behind trigonometry's infamous difficulty, uncovering the allure and intricacies that have captivated mathematicians and learners for centuries.

Historical Background of Trigonometry

Trigonometry's roots can be traced back to ancient civilizations, where the need to understand and apply geometric principles to various practical endeavors spurred the development of rudimentary trigonometric concepts. The ancient Egyptians and Babylonians, renowned for their architectural and astronomical achievements, laid the groundwork for early trigonometric principles through their studies of angles and geometric relationships.

However, it was the ancient Greeks who significantly advanced the field of trigonometry. The mathematician Hipparchus, often hailed as the "father of trigonometry," made substantial contributions to the discipline during the 2nd century BCE. He compiled the first known trigonometric table and applied trigonometric functions to astronomical calculations, marking a pivotal moment in the historical evolution of trigonometry.

The utilization of trigonometric principles continued to evolve throughout history, with Islamic scholars further refining the discipline during the medieval period. Notably, the Persian mathematician Al-Biruni made significant strides in trigonometry, introducing the concept of trigonometric functions and their applications in astronomical studies.

During the Renaissance, trigonometry experienced a resurgence in Europe, with prominent mathematicians such as Johannes Kepler and Isaac Newton incorporating trigonometric concepts into their groundbreaking works on planetary motion and calculus. This period marked a turning point in the widespread acceptance and application of trigonometry as a fundamental tool in scientific and mathematical inquiries.

The historical trajectory of trigonometry reflects its profound impact on diverse fields, from architecture and navigation to astronomy and physics. Its evolution from ancient civilizations to the modern era underscores the enduring relevance and significance of trigonometric principles in shaping our understanding of the natural world and facilitating technological advancements.

In essence, the historical background of trigonometry serves as a testament to the enduring legacy of mathematical inquiry and the intrinsic connection between theoretical concepts and practical applications. The evolution of trigonometry mirrors humanity's quest for knowledge and the timeless quest to comprehend the intricate patterns and relationships that govern the universe.

The Complexity of Trigonometric Functions

Trigonometric functions, such as sine, cosine, and tangent, form the bedrock of trigonometry, embodying a level of complexity that has both intrigued and confounded mathematicians and students alike. At the heart of this complexity lies the intricate interplay between angles, ratios, and circular functions, giving rise to a rich tapestry of mathematical relationships and properties.

One of the defining features of trigonometric functions is their periodic nature, wherein the values of these functions repeat at regular intervals. This periodicity, stemming from the circular nature of trigonometric functions, introduces a layer of intricacy that demands a nuanced understanding of angular measurements and their corresponding function values. The cyclical behavior of trigonometric functions underscores their inherent complexity, requiring learners to grasp the subtle nuances of periodicity and its implications in diverse mathematical contexts.

Furthermore, the transcendental nature of trigonometric functions adds another dimension to their complexity. Unlike algebraic functions, which can be expressed as solutions to polynomial equations, trigonometric functions transcend this framework, giving rise to non-algebraic relationships that challenge conventional mathematical conventions. The transcendental nature of trigonometric functions introduces a level of abstraction and intricacy that necessitates a deeper exploration of their properties and behaviors, presenting both a formidable challenge and an opportunity for intellectual growth.

Another facet of complexity arises from the interconnectedness of trigonometric functions and their profound influence on geometric relationships. These functions serve as fundamental tools for analyzing and modeling periodic phenomena, from the oscillations of pendulums to the undulating patterns of sound waves. The seamless integration of trigonometric functions into diverse scientific and engineering domains underscores their indispensable role in understanding the natural world, underscoring the significance of unraveling their complexities.

In essence, the complexity of trigonometric functions transcends mere mathematical abstraction, permeating various disciplines and real-world applications. Embracing the intricacies of trigonometric functions not only fosters a deeper appreciation for the elegance of mathematical theory but also equips individuals with the analytical tools to comprehend and navigate the complexities of the physical universe.

The enigmatic allure of trigonometric functions lies in their ability to encapsulate profound mathematical truths within their periodic oscillations and transcendental properties, inviting learners to embark on a journey of discovery and mastery. As we unravel the complexities of trigonometric functions, we gain insight into the boundless intricacies of mathematical inquiry and the enduring fascination it holds for those who dare to explore its depths.

The Mysterious Nature of Trigonometric Identities

Trigonometric identities, comprising a diverse array of equations and relationships, possess an enigmatic quality that has captivated mathematicians and learners for centuries. These identities, rooted in the fundamental properties of trigonometric functions, unveil a labyrinth of interconnected patterns and symmetries, offering a glimpse into the profound unity that underlies seemingly disparate mathematical constructs.

At the heart of trigonometric identities lies the intricate web of relationships between sine, cosine, tangent, and other trigonometric functions. These identities manifest in various forms, from fundamental equations like the Pythagorean identity to complex transformations and manipulations that unveil hidden connections between trigonometric expressions. The mysterious allure of these identities stems from their ability to encapsulate profound mathematical truths within seemingly innocuous equations, inviting mathematicians to unravel their cryptic symbolism and extract profound insights.

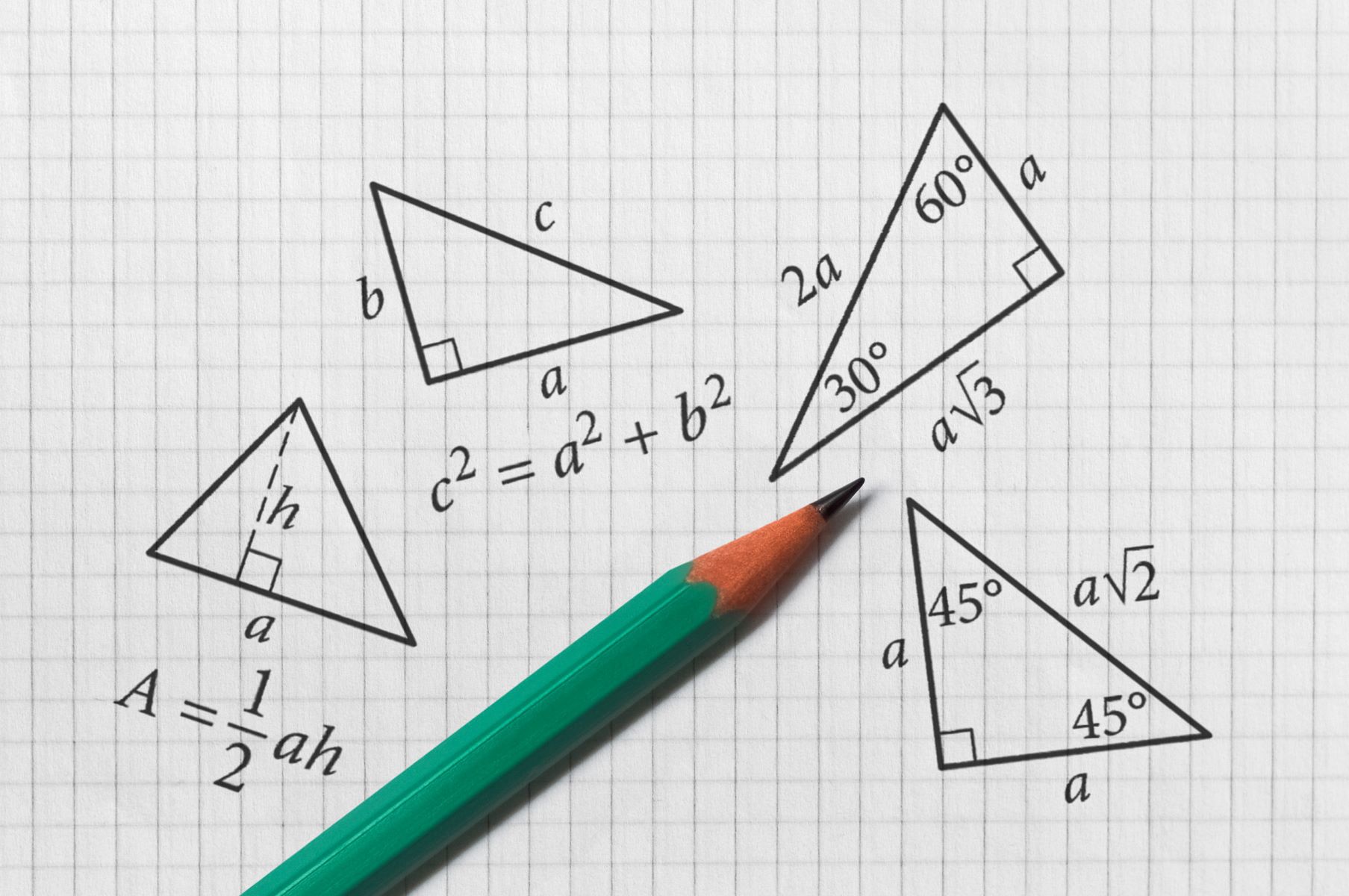
One of the most renowned trigonometric identities, the Pythagorean identity, embodies the elegant simplicity and deep significance that permeates these mathematical constructs. Expressed as sin²θ + cos²θ = 1, this identity unveils a profound relationship between the sine and cosine functions, transcending its seemingly straightforward form to reveal the intrinsic unity between these fundamental trigonometric entities. The Pythagorean identity serves as a cornerstone of trigonometric manipulations, laying the groundwork for diverse mathematical transformations and providing a gateway to deeper explorations of trigonometric relationships.

Moreover, the enigmatic nature of trigonometric identities is further accentuated by their role in diverse mathematical and scientific domains. These identities serve as indispensable tools for simplifying complex trigonometric expressions, solving intricate equations, and uncovering hidden symmetries within mathematical frameworks. From the elegant manipulations of double-angle and half-angle identities to the intricate transformations facilitated by addition and subtraction formulas, trigonometric identities permeate mathematical discourse with their cryptic elegance and transformative power.

As mathematicians and learners grapple with the mysterious nature of trigonometric identities, they embark on a journey of discovery and revelation. The enigmatic allure of these identities lies in their ability to transcend the boundaries of mere equations, offering a glimpse into the profound interconnectedness of mathematical concepts and the timeless elegance that permeates the fabric of trigonometric theory.

In essence, the mysterious nature of trigonometric identities beckons mathematicians to unravel their cryptic symbolism, unveiling the hidden symmetries and profound truths that lie beneath their enigmatic veneer. Through meticulous exploration and contemplation, the intricate tapestry of trigonometric identities unfolds, illuminating the profound unity and interconnectedness that define the timeless allure of mathematical inquiry.

The Role of Trigonometry in Real-World Applications

Trigonometry, often revered for its theoretical intricacies, transcends the realm of abstract mathematics to permeate diverse real-world applications, playing a pivotal role in fields ranging from architecture and engineering to astronomy and technology. The profound significance of trigonometry lies in its ability to provide a powerful framework for analyzing and modeling various natural phenomena, enabling the precise design of structures, the navigation of celestial bodies, and the optimization of technological systems.

In the realm of architecture and engineering, trigonometric principles serve as indispensable tools for designing and constructing structures with meticulous precision. From calculating the dimensions of architectural marvels to determining the optimal angles for load-bearing structures, trigonometry underpins the foundational principles of architectural and structural engineering. The application of trigonometric concepts extends to diverse engineering disciplines, including civil, mechanical, and aerospace engineering, where the understanding of angles, distances, and geometric relationships is essential for realizing innovative designs and engineering solutions.

Moreover, trigonometry finds widespread application in the field of astronomy, where it serves as a fundamental tool for celestial navigation, astronomical measurements, and the study of celestial phenomena. Navigational techniques such as celestial triangulation rely on trigonometric principles to determine the position of celestial bodies and navigate across vast expanses of the sea and sky. Furthermore, astronomers utilize trigonometric parallax to measure the distances to stars and celestial objects, shedding light on the vastness of the cosmos and deepening our understanding of the universe's grandeur.

In the realm of technology and physics, trigonometry plays a crucial role in the design and optimization of various systems and devices. From the precise calibration of optical instruments to the analysis of waveforms in electrical engineering, trigonometric functions and identities form the bedrock of technological innovation. In fields such as telecommunications, signal processing, and robotics, the application of trigonometric principles enables the development of advanced technologies that underpin modern society's interconnected infrastructure.

Furthermore, the pervasive influence of trigonometry extends to disciplines such as geography, cartography, and geodesy, where the measurement and representation of spatial relationships rely on trigonometric concepts. The utilization of trigonometric surveying techniques facilitates the accurate mapping of terrain, the delineation of property boundaries, and the planning of infrastructure projects, contributing to the sustainable development of urban and rural landscapes.

In essence, the role of trigonometry in real-world applications underscores its profound impact on diverse disciplines and its indispensable contribution to technological advancement and scientific inquiry. The seamless integration of trigonometric principles into practical endeavors exemplifies the enduring relevance and significance of this mathematical discipline, illuminating the profound interconnectedness between theoretical concepts and real-world innovation.

Teaching and Learning Challenges in Trigonometry

Teaching and learning trigonometry pose unique challenges that stem from the intricate nature of the subject and the diverse learning styles and mathematical backgrounds of students. The complexities inherent in trigonometric concepts, functions, and identities often present formidable hurdles for both educators and learners, necessitating innovative approaches to instruction and support.

One of the primary challenges in teaching trigonometry lies in conveying abstract mathematical concepts in a tangible and relatable manner. The visual representation of angles, triangles, and periodic functions is crucial for fostering a deep understanding of trigonometric principles. However, translating these abstract concepts into concrete visualizations that resonate with students of varying mathematical proficiencies requires adept instructional strategies and the utilization of interactive tools and resources.

Furthermore, the interconnected nature of trigonometric functions and identities demands a holistic approach to instruction, wherein the relationships between different concepts are elucidated with clarity and coherence. Educators face the challenge of guiding students through the intricate web of trigonometric relationships while addressing individual learning paces and cognitive styles. Tailoring instructional methods to accommodate diverse learning modalities, such as visual, auditory, and kinesthetic approaches, is essential for ensuring comprehensive comprehension and retention of trigonometric principles.

Another significant challenge arises from the application of trigonometry in real-world contexts, where students often encounter difficulties in bridging theoretical concepts with practical applications. Integrating authentic, context-based problems and projects into the curriculum can enhance students' engagement and facilitate a deeper appreciation for the relevance of trigonometry in various fields. However, crafting real-world scenarios that authentically reflect the application of trigonometric principles while aligning with students' experiences and interests requires thoughtful curriculum design and pedagogical creativity.

Moreover, the transition from elementary geometric concepts to the abstract realm of trigonometry poses a cognitive challenge for many learners. The shift from familiar Euclidean geometry to the nuanced study of angles, circular functions, and trigonometric equations demands scaffolding and differentiated instruction to support students in navigating this cognitive leap. Providing ample opportunities for hands-on exploration, collaborative problem-solving, and formative feedback can mitigate the challenges associated with this transition, fostering a supportive learning environment that nurtures mathematical growth and confidence.

In addressing these teaching and learning challenges, educators must cultivate a dynamic and inclusive learning environment that celebrates diverse perspectives and fosters a growth mindset. By embracing innovative instructional practices, leveraging technology-enhanced learning tools, and nurturing a collaborative learning community, educators can empower students to surmount the challenges of trigonometry, instilling a deep appreciation for the elegance and practical significance of this mathematical discipline.

Conclusion

In conclusion, the enigmatic allure and profound significance of trigonometry resonate through its historical legacy, intricate functions, mysterious identities, real-world applications, and teaching and learning challenges. Trigonometry's journey from ancient civilizations to modern scientific and technological frontiers underscores its enduring relevance and transformative impact on diverse domains.

The historical background of trigonometry unveils the timeless quest for understanding geometric relationships and celestial phenomena, reflecting humanity's relentless pursuit of knowledge and innovation. The evolution of trigonometry from ancient civilizations to the Renaissance and beyond highlights its enduring legacy as a foundational pillar of mathematical inquiry and practical applications.

The complexity of trigonometric functions transcends mere mathematical abstraction, permeating various scientific and engineering disciplines with its periodicity, transcendental nature, and profound influence on geometric relationships. Embracing the intricacies of trigonometric functions equips individuals with the analytical tools to comprehend and navigate the complexities of the physical universe, fostering a deeper appreciation for the elegance of mathematical theory.

Trigonometric identities, with their enigmatic symbolism and transformative power, invite mathematicians to unravel their cryptic elegance, unveiling the hidden symmetries and profound truths that lie beneath their enigmatic veneer. The mysterious allure of these identities lies in their ability to transcend the boundaries of mere equations, offering a glimpse into the profound interconnectedness of mathematical concepts and the timeless elegance that permeates the fabric of trigonometric theory.

The role of trigonometry in real-world applications underscores its profound impact on diverse disciplines, from architecture and engineering to astronomy and technology. The seamless integration of trigonometric principles into practical endeavors exemplifies the enduring relevance and significance of this mathematical discipline, illuminating the profound interconnectedness between theoretical concepts and real-world innovation.

Teaching and learning challenges in trigonometry necessitate innovative approaches to instruction and support, addressing the complexities inherent in trigonometric concepts while accommodating diverse learning styles and cognitive backgrounds. By embracing innovative instructional practices, leveraging technology-enhanced learning tools, and nurturing a collaborative learning community, educators can empower students to surmount the challenges of trigonometry, instilling a deep appreciation for the elegance and practical significance of this mathematical discipline.

In essence, the mind-boggling secrets behind trigonometry's infamous difficulty unveil a captivating journey of discovery and mastery, inviting learners to unravel its enigmatic tapestry and embrace the timeless allure of mathematical inquiry. As we conclude this exploration, the enduring legacy and profound significance of trigonometry resonate as a testament to humanity's enduring quest for knowledge, innovation, and the timeless beauty of mathematical theory.